how to find asymptotes of a function
Asymptotes: Examples
So far, we've dealt with each type of asymptote separately, kind of like your textbook probably does, giving one section in the chapter to each type. But on the test, the questions won't specify which type you need to find.
In general, you will be given a rational (fractional) function, and you will need to find the domain and any asymptotes. You'll need to find the vertical asymptotes, if any, and then figure out whether you've got a horizontal or slant asymptote, and what it is. To make sure you arrive at the correct (and complete) answer, you will need to know what steps to take and how to recognize the different types of asymptotes.
Let's get some practice:
Content Continues Below
-
Find the domain and all asymptotes of the following function:
I'll start with the vertical asymptotes.
They (and any restrictions on the domain) will be generated by the zeroes of the denominator, so I'll set the denominator equal to zero and solve.
Then the domain is all x -values other than , and the two vertical asymptotes are at .
Next I'll turn to the issue of horizontal or slant asymptotes.
Since the degrees of the numerator and the denominator are the same (each being 2), then this rational has a non-zero (that is, a non- x -axis) horizontal asymptote, and does not have a slant asymptote. The horizontal asymptote is found by dividing the leading terms:
Then the full answer is:
domain:
vertical asymptotes:
horizontal asymptote:
slant asymptote: none
A given rational function may or may not have a vertical asymptote (depending upon whether the denominator ever equals zero), but (at this level of study) it will always have either a horizontal or else a slant asymptote.
Note, however, that the function will only have one of these two; you will have either a horizontal asymptote or else a slant asymptote, but not both. As soon as you see that you have one of them, don't bother looking for the other one.
-
Find the domain and all asymptotes of the following function:
The vertical asymptotes come from the zeroes of the denominator, so I'll set the denominator equal to zero and solve.
Oops! This has no solution. (Duh! The denominator is a sum of squares, not a difference. So of course it doesn't factor and it can't have real zeroes. I should remember to look out for this, and save myself some time in the future.)
Since the denominator has no zeroes, then there are no vertical asymptotes and the domain is "all x ".
Since the degree is greater in the denominator than in the numerator, the y -values will be dragged down to the x -axis and the horizontal asymptote is therefore " y = 0". Since I have found a horizontal asymptote, I don't have to look for a slant asymptote.
My full answer is:
domain: all x
vertical asymptotes: none
horizontal asymptote: y = 0 (the x -axis)
slant asymptote: none
The Special Case with the "Hole"
We've dealt with various sorts of rational functions. When you were first introduced to rational expressions, you likely learned how to simplify them. You'd factor the polynomials top and bottom, if you could, and then you'd see if anything cancelled off.
What if you've found the zeroes of the denominator of a rational function (so you've found the spots disallowed in the domain), but one or another of the factors cancels off? Let's look at an example of exactly that situation:
-
Find the domain and all asymptotes of the following function:
It so happens that this function can be simplified as:
So the entire rational function simplifies to a linear function. Clearly, the original rational function is at least nearly equal to y = x + 1 — though I need to keep in mind that, in the original function, x couldn't take on the value of 2. But what about the vertical asymptote? Is there one at x = 2, or isn't there?
If there is a vertical asymptote, then the graph must climb up or down it when I use x -values close to the restricted value of x = 2. I'll try a few x -values to see if that's what's going on.
x = 1.5, y = 2.5
x = 1.9, y = 2.9
x = 1.95, y = 2.95
x = 1.99, y = 2.99
Not only is this not shooting off anywhere, it's actually acting exactly like the line y = x + 1. So apparently the zero of the original denominator does not generate a vertical asymptote if that zero's factor cancels off.
While the graph of the original function will look very much like the graph of y = x + 1, it will not quite be the same. And, whether or not I'm graphing, I'll need to remember about the restricted domain.
Since the degree of the numerator is one greater than the degree of the denominator, I'll have a slant asymptote (not a horizontal one), and I'll find that slant asymptote by long division.
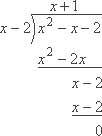
Hmm... There wasn't any remainder when I divided. Actually, that makes sense: since x – 2 is a factor of the numerator and I'm dividing by x – 2, the division should come out evenly. And, as I'd kind-of expected, the slant asymptote is the line y = x + 1.
Then the full answer is:
domain: x ≠ 2
vertical asymptotes: none
horizontal asymptote: none
slant asymptote: y = x + 1
This last case ("with the hole") is not the norm for slant asymptotes, but you should expect to see at least one problem of this type, including perhaps on the test.
By the way, when you go to graph the function in this last example, you can draw the line right on the slant asymptote. But you will need to leave a nice open dot (that is, "the hole") where x = 2, to indicate that this point is not actually included in the graph because it's not part of the domain of the original rational function.
To summarize, the process for working through asymptote exercises is the following:
- set the denominator equal to zero and solve (if possible)
- the zeroes (if any) are the vertical asymptotes (assuming no cancellations)
- everything else is in the domain
- compare the degrees of the numerator and the denominator
- if the degrees are the same, then you have a horizontal asymptote at y = (numerator's leading coefficient) / (denominator's leading coefficient)
- if the denominator's degree is greater (by any margin), then you have a horizontal asymptote at y = 0 (the x -axis)
- if the numerator's degree is greater (by a margin of 1), then you have a slant asymptote which you will find by doing long division
The only hard part is remembering that sometimes a factor from the denominator might cancel off, thereby removing a vertical asymptote but not changing the restrictions on the domain. You might even want to get in the habit of checking if the polynomials in the numerator and denominator factor, just in case.
Either way, when you're working these problems, try to go through the steps in order, so you can remember the whole process on the test. These exercises are not so hard once you get the hang of them, so be sure to do plenty of practice exercises.
how to find asymptotes of a function
Source: https://www.purplemath.com/modules/asymtote4.htm
Posted by: mcquadefrouss.blogspot.com

0 Response to "how to find asymptotes of a function"
Post a Comment